|
|
|
{Page 81}
Department of Mechanical Engineering, University of Tennessee, Knoxville, Tennessee, and
School of Aerospace, Mechanical and Nuclear Engineering, University of Oklahoma, Norman, Oklahoma
Four different surfaces can be identified in the vicinity of the axis of a tornado — the observed funnel cloud, the surface of maximum swirling velocity, the condensation pressure isobar and the boundary of the recirculating core region. Arguments are presented to suggest that these four surfaces can be, and in some cases are, distinct. An estimate of the radius of maximum swirling velocity is developed on the basis of a flow model that ignores viscous forces. This inviscid estimate gives a radius that is typically much larger that that derived from viscous-structured vortex models and which is in agreement with Hoecker's observations for the 2 April 1957 Dallas tornado. By means of a diffusion-limited model of the condensation process, the observed funnel cloud is shown to lie within the condensation pressure isobar. Depending upon parameter values, the distance between these two surfaces can be rather large, and, as a consequence, cause errors in the maximum wind estimates made from photographic evidence.
We wish to propose a conceptual model of tornado flows near the tornado axis in which four distinct surfaces are identified — the surface of maximum tangential velocity, the observed funnel, the condensation pressure isobar, and the boundary of the recirculating core (see Figure 1). While there is a paucity of data for naturally occurring tornadoes, Hoecker's (1, 2) observations of the 2 April 1957 Dallas tornado do provide an example of a tornado in which the surface of maximum tangential velocity, the observed funnel, and condensation pressure isobar are unquestionably distinct. In this regard, laboratory simulations (see e.g. refs. 3, 4, 5, and 6) are of limited utility as they do not adequately model the condensation process. The "funnels" observed in those simulations are, ignoring diffusion, streamsurfaces. Laboratory simulations of tornado flows, thus, are likely to be of little use in examining the relationship between the funnel clouds observed in naturally occurring tornadoes and the recirculating core flow (if such exists). While the intense swirling motion imposed in these simulations makes the observed streamsurface superficially similar to the funnel cloud of a naturally occurring tornado, one should not assume that the two surfaces are identical. As a consequence, discussions of tornado funnel clouds must rely on the available observations of naturally occurring tornadoes and approximate calculations based on the dynamic and thermodynamic processes in these vortex flows.
In order to describe the observed features of naturally occurring tornadoes, we believe that a clear distinction must be made between four different funnel-like surfaces in the vicinity of the tornado axis (see Figure 1). This is important if realistic estimates of the significant parameters of the flow are to be obtained — especially near the axis of the tornado — and if the
{Page 82}
wide variety of observations are to be reconciled.
The first surface — the most obvious feature of naturally occurring tornadoes — is the observed trunk-shaped funnel cloud which is pendant from the cloud deck of the storm. The size, behavior and motion of the funnel can be determined relatively easily by photographic methods. Although this funnel is the most readily observed feature of tornadoes, there is uncertainty as to what it represents. The most common assumption is that the tornado funnel is the condensation pressure isobar. This is based, in part, on the similarity of the isobaric surfaces and the funnel shape for strongly swirling flows (7). A second possible assumption is that the funnel is a streamsurface (2) — e.g., there is no flow normal to the observed surface of the funnel.
The second surface of interest is the cylindrical surface generated by the radius rm (z) where the maximum tangential velocity occurs. If one assumes the cyclostrophic balance between the radial pressure gradient and centripetal acceleration holds, then the maximum pressure drop in a tornado is proportional to rm-2. The value of rm is thus of great practical interest in assessing the loads on structures in tornadic winds. Hoecker's (1) observations allow rm (z ) to be determined for the 1957 Dallas tornado (see Figure 2). As we shall demonstrate later in detail, Hoecker's results for rm are considerably larger than those which would be inferred by viscous-structured vortex models — that is, models in which the radius of the maximum winds is determined by a balance between outward radial diffusion of angular momentum and inward convection of angular momentum. It is also worth noting that in the case of the Dallas tornado, this relatively large-diameter, quasi-cylindrical surface r = rm (z) begins from the ground surface and is distinct from the tornado funnel cloud throughout the period of observation, in part as a consequence of the hydrostatic contribution to the pressure.
The third surface of interest in the condensation pressure isobar (p = pc ) given by the conditions far away from the tornado vortex. Because of the intense swirling motion, the shape of this surface, which is similar to that of other pressure isobars, resembles the observed funnel cloud and is commonly assumed to be coincident with it. Yet in the one case where this assumption can be tested, namely the 1957 Dallas tornado, it is found to be invalid. The diameter of the condensation pressure isobar, derived by Hoecker from the measured velocity field and inferred pressure distribution using a cyclostrophic assumption, was larger (at all heights) than the diameter of the tornado. We believe that Hoecker's observations can be explained by considering the details of the time-dependent condensation process in the converging, swirling flow outside the funnel cloud.
The fourth funnel-like surface in tornadoes is the boundary of the recirculating core of the tornado. This is the boundary that divides the vortex flow into two regions — an outer, rising, converging swirling flow region and an inner, recirculating core region. The assumption of a recirculating core region presupposes the existence of a downflow along the vortex axis. Although not a certainty, there exists supporting evidence for a downflow in the
{Page 83}
core of real tornadoes (2) as well as in the laboratory simulation models (6). The existence of an exact solution to the Navier-Stokes equation for a vortex flow with a double cell structure (8), experimental evidence (9), and the assumed nature of the conditions aloft (imposed constant pressure boundary) have led several authors to assume a descending flow on the axis of the core. It should be noted, however, that the existence of downflow along the axis would not necessarily imply a closed recirculating core flow. In fact, the nature of the closure aloft still remains a difficult question to be resolved.
Thus it appears that four distinct surfaces can be identified in the vicinity of the tornado axis. While this description is motivated in part by the Hoecker results for the Dallas 1957 tornado clearly one cannot justify a "model" of tornado, vortices on one observation. Rather, we view the Hoecker results as suggesting the need for distinguishing these four funnel-like surfaces when discussing tornado flows. In what follows, we shall present theoretical estimates which support the contention that the Hoecker observations are not unique and a four-surface model is consistent with the constraints imposed by the principles of fluid dynamics and thermodynamics.
Some investigators (e.g. refs. 7 and 10) have assumed that the observed funnel of a tornado, the condensation pressure isobar and, in some cases, the streamsurface bounding the recirculating core are all coincident. We now want to show that although the observed funnel is a condensation surface, it need not coincide with the condensation pressure isobar in the vicinity of the tornado axis.
The tornado funnel would coincide with the condensation pressure isobar only if the characteristic time for condensation to occur is much smaller than the characteristic flow time of air particles — the time required for a fluid particle to traverse a characteristic length. That is, if the former time is tc and the latter tf, then a nondimensional parameter k can be defined as k = tc/tf. If k < < 1, the tornado funnel is expected to coincide with the condensation isobar. This limiting case is the "equilibrium" situation in which the condensation process proceeds much faster than the typical time scale of motion of the particles. On the other hand, if k > > 1, then the tornado funnel is expected to coincide with the tornado core. This corresponds to the so called "frozen" situation in which the condensation process is relatively very slow. Presumably a funnel cloud occurs in the case k > > 1 because water vapor is brought down in the core from the cloud deck above by means of the recirculating flow in the core. Hoecker's (1) data and his interpretation of it suggest that the Dallas tornado was close to this limiting "frozen" case.
The time required for a nucleus to grow to an observable radius by means of the condensation process is dependent on many factors such as pressure, water vapor content, etc., but the order of magnitude of the characteristic time is 1-10 seconds even for large (50%) pressure decreases. This relatively large condensation time results because of the slow diffusion process which is the limiting factor in the condensation process.
We now want to estimate the order of magnitude of the condensation time for the particular case of the Dallas tornado. The condensation pressure at the time of the tornado was 950 mb. (2). The growth of a spherical drop as a consequence of diffusion is determined by (11)
 |
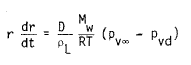Eq. 1 |
where r, D, rL, MW, R, T, pv¥ , pvd are the radius of the drop, diffusion coefficient for water vapor in air, liquid water density, molecular weight of water, universal gas constant, ambient temperature, water vapor pressure far away from the drop, and vapor pressure at the surface of the drop, respectively. Here we have ignored the effects of droplet convection and heat transfer. If the initial radius of the drop is ro and the final radius rf, we obtain from Eq. 1
 |
Eq. 2 |
{Page 84}
If we take the following as being typical parameter values
 |
(e.g. an observable cloud particle must have a radius of ten microns or more), Eq. 2 can then be used to estimate the order of magnitude of the condensation time tc in seconds as
 |
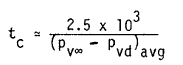Eq. 3 |
if pressure is measured in newtons/m2. Thus, depending upon the average value of (pv¥ - pvd ) the characteristic time for condensation can be large or small.
For the case of the Dallas tornado, we chose a streamline passing through a point at approximately 150 meters radius from the tornado axis with a height of 20 meters from the ground (1). Using Hoecker's (2) derived pressure distribution, the pressure variation on this streamline was determined (see Figure 3). Assuming dry adiabatic cooling, we also determined the temperature history of the air parcels on this streamline (see Figure 4). For T = 283 K, a maximum temperature change of 10 degrees is obtained. Thus, using the temperature history shown in Figure 4, we obtain pv¥ 
1170 n/m2 and pvd 967 n/m2. Hence, an approximate evaluation of Eq. 3 for the Dallas tornado yields tc 1.2 seconds. The assumptions made in obtaining this estimate suggest that an exact calculation would give a somewhat larger result. However, a more detailed droplet-growth model should not change the order-of-magnitude estimate of tc drastically.
For tc of the order of one second or more, a fluid parcel can travel a distance well over fifty meters (at velocities of the order of fifty meters per second) after it has crossed the condensation pressure isobar before visible condensation occurs. These distances are comparable to the radius of the condensation pressure isobar! Hence, given the velocity fields of typical tornadoes, the time-dependent nature of the condensation process in naturally occurring tornadoes can be of consequence. Once an air parcel's pressure is reduced to the condensation pressure, the parcel may travel distances of the order of a hundred meters before visible condensation has occurred. Thus the observed funnel cloud and condensation pressure isobar need not coincide. Furthermore, the recirculating region and
{Page 85}
— in the case of a funnel which does not reach the ground — the stagnation point near the tip of the funnel should always lie on or within the funnel cloud if saturation conditions are achieved in the outer flow. Under these conditions, we expect that the recirculating core flow will be enclosed by the condensation surface of the tornado funnel cloud.
The existence of a time-dependent condensation process also implies that the position of the tip of the observed funnel could be displaced a considerable distance from the corresponding point on the condensation isobar due to the large vertical velocities on the axis of the tornado. In this regard, Dergarabedian and Fendell (7) obtain estimates of maximum tangential velocity based on the distance of the funnel tip from the ground assuming that the funnel surface is coincident with the condensation pressure isobar. However, if the associated condensation parameter k is not small, such estimate could be in error. The diffusion-growth model implies that the "tip" of the p = pc isobar is below the funnel tip by a distance equal to approximately Wctc where Wc is the average vertical velocity on the axis. For example, for the Dallas tornado — where the funnel cloud tip was in mid-air for a considerable portion of the observed life of the tornado — Wc 50 m/sec (see Figure 5). Thus, the difference in height between the condensation pressure isobar and funnel tip could amount to 50 m causing an underestimation of the maximum velocity. However, if the actual value of tc tends toward the lower end of possible values, (e.g. tc 0.1 sec) this difference is reduced to about 5 m and the resulting error is less than ten percent.
Whether nonequilibrium condensation effects are important in a particular tornado depends on such variable factors as the size of the soluble condensation nuclei present, the magnitude of the wind speed, and so on. This discussion does show, however, that there can be circumstances in which the observed funnel and condensation pressure isobar are quite distinct and other circumstances in which they coincide. One could attribute, at least in part, the wide variation in the appearance of tornado funnels to this range of circumstances. Smooth-walled funnels could correspond to the fast condensation case (k ® 0) in which the funnel is an isobar. Rough-walled funnels then may corespondend to the slow condensation case (k ® ¥) where the turbulence in the recirculating core gives the funnel its rough appearance.
It is clear that an exact analysis of a tornado vortex flow cannot ignore the effects of viscous forces. This does not imply, however, that the radius of maximum tangential velocity is determined by viscosity as suggested by the viscous vortex models (8, 12, 13). If one estimates the radius of maximum tangential velocity (r = rm) from these viscous-structured vortex models, results are obtained which are too small by a factor of from three to six when compared with Hoecker's data for the 1957 Dallas tornado. And these estimates use a value of the eddy viscosity (30 m2/s2) which is rather large. Although a coupling of the meridional and tangential flows by viscosity might be important in describing the observed variation of tangential velocity with height (giving, in turn, rm = rm (z) ), we propose that the dimensions of the core are determined by flow field dynamics that are essentially inviscid.
Let pa (z) be the pressure along the axis of the cylindrical region bounded by the surface of maximum tangential velocity. We can estimate pa (z) in two ways. First, the inviscid momentum equation can be integrated along the axis of the tornado (e.g. along the streamlined r = 0) to give Bernoulli's equation,
 |
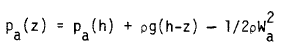Eq. 4 |
where the height h is the point on the axis where the vertical velocity Wa vanishes. Assuming the cyclostrophic balance holds and that the tangential velocity is that of a combined Rankine vortex (see e.g. refs. 1 and 14), we also obtain
 |
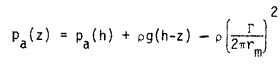Eq. 5 |
where p¥ (z) (= pw(h) + r g(h-z)) is the ambient pressure distribution far from the tornado axis (r >> rm ) where the velocity is negligible and G is the circulation
{Page 86}
of the velocity far from the tornado axis. Comparing Eq. 4 and 5 we have
 |
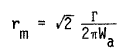Eq. 6 |
which provides an estimate for the radius of maximum tangential velocity. Assuming a nonrotating core as suggested by Davies-Jones (15) removes the 
factor in Eq. 6. As this estimate is based on an inviscid analysis, we should note that Eq. 6 is not valid in the immediate vicinity of the ground where Ww ® 0, G ® 0 and viscous forces are important.
We can compare this result with the Hoecker data. Choosing a streamline with G = 220 m2 /sec and an axial velocity of 45 m/sec at z = 120 meters, we obtain rm = 60 meters. This is in good agreement with the observed values (see Figure 2) especially when one considers the approximate nature of the calculation. In contrast, viscous-structured vortex models give estimates for rm ranging from 10 to 20 meters. We conclude that an inviscid analysis based on a pressure-matching condition gives an estimate of the radius where the maximum tangential velocity occurs which is in much better accord with observations than those derived from viscous-structured vortex models.
It should also be noted that this analysis implies the axial velocity is the same order of magnitude as the tangential velocity near the axis. Since V = G /2pr, Eq. 6 implies Vmax and Wa are of the same order of magnitude. Hoecker's measurements of axial and swirling velocity fields (1) substantiate this conclusion (see Figure 5). Qualitative observations of the core flow in Ward's simulator are also in agreement (9).
Finally, for the sake of completeness, we wish to demonstrate that the surface of maximum tangential velocity (r = rm) and the funnel cloud do not coincide. To do so, we shall assume that the funnel cloud is approximately given by a pressure isobar p1 (corresponding to the near-equilibrium condensation limit k ® 0) and the tangential component of velocity field is given by a combined Rankine vortex. Assuming a cyclostrophic balance, we obtain
 |
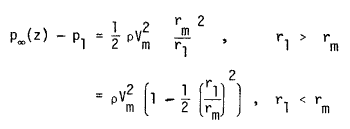Eq. 7 |
where p¥ (z) (= p¥ (0) r gz) is the ambient pressure far from the tornado axis and Vm (z) is the maximum tangential velocity. We observe that, depending upon the value of (p¥ (z) – p1)/r Vm (z)2 relative to 1/2, the funnel can lie outside or inside the radius of the maximum tangential velocity. Specifically,
 |
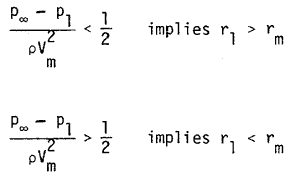Eq. 8 |
The ambient pressure p¥ (z) far from the tornado axis has its maximum value near the ground. Thus, Eq. 8 suggests that the tornado funnel should have its minimum diameter near the ground and the diameter should increase with height — assuming Vm (z) is roughly constant. Indeed, since p¥ (z) decreases with height, the funnel could lie inside rm far near the ground and outside rm far above the ground. Of course, if Vm (z) decreases with height, the funnel diameter could increase more slowly, remain constant, or decrease with height.
Since, in addition, p1 is determined by factors such as the water content of the surface layer of the atmosphere which would seem to be independent of the factors that determine p¥ (z) and Vm (z)2, Eq. 8 implies that the tornado funnel cloud could lie inside or outside or cross the surface of maximum tangential velocity. Hoeckers (2) data confirm the existence of at least the former possibility. In this regard, the unusual observations of the Ellsworth, Kansas, tornado of June 20, 1926 (16) suggests that for sufficiently low p1 (very dry air in the lower atmosphere) the vortex could fail to produce a funnel. While the photographic evidence does not show a funnel, the debris pattern on the ground nonetheless does suggest the existence of a vortex there.
{Page 87}
A schematic of the four surfaces in the vicinity of the tornado axis is sketched in Figure 5. Were it not for the paucity of data on the wind fields of naturally occurring tornadoes, this proposed structure could be tested more carefully. The absence of data, however, makes conclusions concerning the ultimate flow structure speculative. Nonetheless, the analysis and discussion does begin to suggest that the usual assumptions concerning the structure of tornado vortex flows need not be true and that the wide variations in tornado funnel appearance may be understandable. Finally, the utility of laboratory simulations of tornado vortices can be more carefully assessed now that the possible distinctiveness of the surface of maximum swirling winds and the funnel cloud surface is recognized.
1. W. H. HOECKER, Mon. Weather Rev. 88: 167-180 (1960).
2. W. H. HOECKER, Mon. Weather Rev. 89: 533-542 (1961).
3. S. J. YING and C. C. CHANG, J. Atm. Sci. 29: 3-14 (1972).
4. C. A. WAN and C. C. CHANG, J. Atm. Sci. 29: 116-127 (1972).
5. J. S. TURNER, J. Fluid Mech. 25: 377-400 (1966).
6. N. B. WARD, J. Atm. Sci. 29: 1194-1204 (1972).
7. P. DERGARABEDIAN and F. FENDELL, J. Aeronaut. Sci. 17: 215-236 (1970).
8. R. D. SULLIVAN, J. Aero/Space Sci. 26: 767-768 (1959).
9. M. PARANG, Fluid Dynamics of a Tornado-Like Vortex Flow, Ph.D. Dissertation, University of Oklahoma, Norman, 1975.
10. A. H. GLASER, in: C. E. ANDERSON (ed.), Cumulus Dynamics, Pergamon Press, London, 1960, pp. 157-166.
11. B. J. MASON, The Physics of Clouds, Clarendon Press, 2d Edition, Oxford, 1971, pp. 122-125.
12. N. ROTT, Z. ANGEW. Math. Phys. 9: 543-553 (1958).
13. H. L. KUO, J. Atm. Sci. 25: 20-42 (1971).
14. M. C. JISCHKE and M. PARANG, J. Atm. Sci. 31: 506-512 (1974).
15. R. P. DAVIES-JONES, J. Atm. Sci. 30: 1427-1430 (1973).
16. S. D. FLORA, Tornadoes of the United States, University of Oklahoma Press, Norman, 1959.
