
|
 |
|
{Page 72}
School of Aerospace, Mechanical, and Nuclear Engineering, University of Oklahoma Norman, Oklahoma 73019
| Top of Page | References | Table of Contents | Home |
Jischke (1) has proposed a fluid-dynamic model of the fault slip zone between underthrusting oceanic crust and overriding continents as a thin fluid layer in which heating occurs because of viscous dissipation. It differs from the one-dimensional Couette flow midel of Turcotte and Oxburgh (2) by allowing the thickness of the slip zone to vary with depth. An important consequence of this variable slip-zone thickness is that the slip zone subjects the descending lithospheric slab to both a shear force and a nonhydrostatic pressure force which can balance the gravitational force acting on the slab. In this way, the descending plate adheres to the overriding block, maintaining an angle of descent dictated by the geometry of the overriding continent. Thus the observed constancy of the dip angle of descending plates can be explained. In addition, this theoretical model allows one to estimate the slip zone thickness, viscosity, shear stress and energy dissipation. The results obtained are in good agreement with accepted values.
The model does appear to have one major weakness: it cannot explain the observed relationship between the velocity of descent of the lithospheric plate and the dip angle. Table 1 below gives results for the velocity and dip angle of several different subduction zones. Here U is the subduction velocity normal to the trench and q is the seismically determined dip angle, measured from the downward vertical. The values for U are from the results presented by Solomon, Sleep, and Richardson (3). We have not included the Marianas trench as it does not appear to have an overriding block to which it can adhere and thus is not described by the present model.
Jischke's model, which assumes the descending plate is in mechanical equilibrium under the action of gravitational, pressure, and viscous shear forces, gives
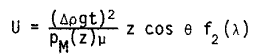 |
Eq. 1 |
Here Dr is the density contrast between the descending slab and the mantle, g is the acceleration of gravity, t is the slab thickness, z is the depth to which the slab sinks (roughly 100 km), PM(z) is the mantle pressure at the depth z, µ is the assumed constant viscosity of the slip zone fluid, and l is the constant of proportionality in the assumed linear variation of (nondimensional) slip zone thickness with distance along the zone. The function f2 is weakly dependent on l, varying from 0.5 to 0.35 as l varies from 0 to 10. In addition, we do not expect Dr, g, t, z, or
{Page 73}
PM (z) to be related to the dip angle of the slab. Hence, if the slip zone viscosity µ is constant, Eq. 1 implies U varies as cos q — i.e., U decreases with increasing q. This variation is compared with the data in Figure 1 where it is seen that not only is there poor numerical agreement, but even the trend of the data does not agree with the theory. Indeed, the measurements seem to suggest roughly that the plate velocity increases with the dip angle.
Thus, we have the rather curious situation of a theoretical model which gives good agreement on several comparisons with observations — slip zone thickness, viscosity, shear stress, and dissipation — and, at the same time, completely misses what may be the most important variable — the plate velocity. It is becoming clear that once the plate velocities are known, rather simple fluid dynamic models using these velocities as boundary conditions can reproduce many of the features of the mantle including the observed dip angles (e.g. Hager and O'Connell (13) ). The fundamental question then becomes: what determines the plate velocities? It is our contention that the velocity of a plate is determined by the dynamics in the trench slip zone. Of the forces driving the global motion, those acting on the descending slab are far and away the largest (see e.g. Forsyth and Uyeda (14) and Solomon and Sleep (15) ). These forces — gravity, pressure, and viscous — must thus be in mechanical equilibrium, at least approximately. In our view, this condition of mechanical equilibrium determines the resulting plate velocity.
Consequently, Eq. 1 should describe the variation of the plate velocity with q. We believe it does, in fact, give U as a function of q provided one takes account of the fact that the viscosity µ of the slip zone varies with q. Several mechanisms can be postulated within the framework of the thin slip-zone model that cause a variation of µ with q . For example, viscous dissipation (which increases with velocity U) can cause internal heating that changes the average slip-zone temperature and thus the viscosity. Analysis shows, however, that this effect tends to cause the plate velocity to decrease even more rapidly with q than the theoretical result shown in Figure 1. A second mechanism might be found in the varying amounts of oceanic sediments that are entrained into the slip zone. If one assumes that the amount of sediment entrained is proportional to the slip-zone thickness and that increasing the relative amount of oceanic sediments in the slip-zone decreases the average viscosity, then this mechanism will also cause the plate velocity to decrease more rapidly with q than the theoretical result shown in Figure 1.
A third possibility that we favor attributes the variation of µ with q to the involvement of water which is released from the descending slab. As we shall see, the associated variation of µ with q is sufficiently strong that the cos q variation implied by Eq. 1 when µ is assumed constant is quite misleading. Indeed, water involvement allows the trend of U with q to be reversed.
We propose that the source of most of the water is dehydration of the oceanic crust during underthrusting. Anderson, Uyeda, and Miyashiro (16) suggest that the upper part of the oceanic crust contains 3.5% water by weight in a metabasalt layer 2 km thick. This water is released by endothermic dehydration reactions. These reactions represent a significant heat sink for the slab and slip zone and thus buffer the temperature field in that zone as well
{Page 74}
as provide a source of water for the slip zone. The amount of water that enters the slip zone is presumably determined by temperature and pressure distributions within the downgoing slab as well as various physical properties of the slab and the water (e.g. slab porosity, water viscosity, etc.). Since there is a pressure gradient across the slab as a consequence of the nonhydrostatic pressure variation in the slip zone and the roughly hydrostatic variation in the mantle, we propose that water enters the slip zone from the slab at a rate determined by the pressure gradient across the slab. For simplicity, we further assume a simple linear relation between the mass flux and the pressure gradient — i.e., Darcy's law,
 |
Eq. 2 |
Here the subscript w refers to water. The average velocity of the water as it percolates through the slab is vW, k is the permeability of the slab, µW is the dynamic viscosity of the water, and D P is the pressure drop across the slab of thickness t.
To determine the amount of water entering the slip zone from the underthrusting slab, consider a differential element of oceanic crust of volume t D A (D A is the surface area of the element) as it enters a trench. Let us assume that hydrated minerals are found in a layer of thickness d and that upon complete dehydration, the mass fraction of water in the layer is a. Then if b is the fraction of this water driven out of the differential element of crust by pressure forces during the time it takes the element to travel the length of the slip zone (i.e., z/U cos q ), we have
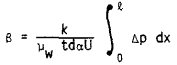 |
Eq. 3 |
where ![]() is the plate length (
is the plate length (![]() = z/cos q ). The integral in Eq. 3 gives the net pressure force (per unit width) acting on the slab and thus must be equal to the component of the gravity force (per unit width) acting on the slab — D r
= z/cos q ). The integral in Eq. 3 gives the net pressure force (per unit width) acting on the slab and thus must be equal to the component of the gravity force (per unit width) acting on the slab — D r![]() tg sin q — if the slab is in mechanical equilibrium. Thus
tg sin q — if the slab is in mechanical equilibrium. Thus
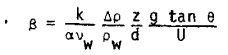 |
Eq. 4 |
If we take the following as nominal values,

we obtain b = 0.6 tan q, suggesting that there is a wide range of values of q for which only a fraction of the water enters the slip zone. More importantly, b is predicted to increase with q. Since the viscosity of mantle material decreases with increasing water involvement, we thus find that the viscosity µ in the slip zone decreases with increasing q. Tsukahara's (17) work shows that the effective viscosity varies exponentially with the mass fraction of water. Water, through its effect on viscosity, can thus have a marked effect on the dynamics of slip zones. Consequently, the trend of U with q as implied by Eq. 1 with µ constant is likely to be in error.
To further illustrate the effect of water involvement on the crustal velocity, we assume the viscosity µ is exponentially dependent on the average mass fraction of water in the slip zone,
| Eq. 5 |
where e is a constant. Further assuming that the only variation of b with q is due to the combination U/tan q, we can rewrite Eq. 1 as
| Eq. 6 |
where a and g are constants. Choosing a nominal condition to evaluate the constant a (here taken to be the observed conditions for the Kurile trench), we can rewrite Eq. 6 as
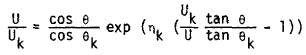 |
Eq. 7 |
where hk = g tan qk /Uk is the only parameter that survives. This implicit equation for U/Uk as a function of q has been solved numerically and the results are compared with observations in Figure 2 for different values of hk. While not all of the trench data agree with Eq. 7, most of the data appear to follow the trend given by that equation with hk of the order of unity. Importantly, the observed trend of increasing U with q is reproduced.
{Page 75}
The singular behavior implied by Eq. 6 as q approaches p/2 is artificial and can be eliminated by noting that the maximum value of b , the fraction of water driven out of the descending plate, is unity. Thus, beyond a critical value of q , given by
 |
Eq. 8 |
all the available water is driven out of the descending slab. For values of q beyond this critical value, the viscosity no longer decreases with increasing q but remains roughly constant. Thus U does not grow without limit as q approaches p/2.
We conclude that inclusion of the effects of water involvement, especially at it affects the viscosity of the slip zone, is a potentially important part of an adequate description of the dynamics of trench systems. Further, we believe that the velocity of crustal plate movements is determined by forces acting in the slip zone and can be estimated using the model described herein. However, more accurate numerical estimates will require more precise information regarding the effect of water on the slip zone viscosity as well as the porosity and permeability of underthrusting oceanic crust.
The author acknowledges discussions with A. Shehadeh, J. Ahern, and J. Wickham of the University of Oklahoma. S. Babb and G. Atkinson of the University of Oklahoma graciously provided data on the viscosity of water at high pressures and temperatures.
| Top of Page | References | Table of Contents | Home |
1. M. C. JISCHKE, J. Geophys. Res. 80: 4809-4813 (1975).
2. D. L. TURCOTTE and E. R. OXBURGH, Phys. Earth Planet. Inter. 1: 381-386 (1968).
3. S. C. SOLOMON, N. H. SLEEP, and R. M. RICHARDSON, Geophys. J. R. Astron. Soc. 42: 769-801 (1975).
4. E. R. ENGDAHL, N. H. SLEEP and M. T. LIN, Tectonophysics 37: 95-116 (1977).
5. J. W. DEWEY and S. T. ALGERMISSEN. Bull. Seismol. Soc. Am. 64: 1033-1048 (1974).
6. B. L. ISACKS and M. BARAZANGI, in: M. TALWARN and W. C. PITMAN III (eds.), Island Arcs, Deep Sea Trenches and Back-Arc Basins, Maurice Ewing Ser., Vol. 1, Washington, D.C., 1977, pp. 99-114.
7. S. UYEDA and H. KANAMORI, J. Geophys. Res. 84: 1049-1061 (1979).
8. T. FITCH, Bull. Seismol. Soc. Am. 60: 565-591 (1970).
9. B. ISACKS and P. MOLNAR, Rev. Geophys. Space Phys. 9: 103-174 (1971).
10. S. N. COHN, unpublished thesis, Harvard College, Cambridge, MA (1975).
11. T. J. FITCH and P. MOLNAR, J. Geophys. Res. 75: 1431-1444 (1970).
12. J. H. ANSELL and E. G. C. SMITH, Nature 253: 518-520 (1975).
13. B. H. HAGER and R. J. O'CONNELL, Tectonophysics 50: 111-133 (1978).
14. D. FORSYTH and S. UYEDA, Geophys. J. R. Astron. Soc. 43: 163-200 (1975).
15. S. C. SOLOMON and N. H. SLEEP, J. Geophys. Res. 79: 2557-2567 (1974).
16. R. N. ANDERSON, S. UYEDA, and A. MIYA-SHIRO, Geophys. J. R. Astron. Soc. 44:333-357 (1976).
17. H. TSUKAHARA, J. Phys. Earth 22: 343-358 (1974).
| Top of Page | References | Table of Contents | Home |