
|
 |
|
{Page 43}
| Introduction | Example 1 | Example 2 | Example 3 | Other Caveats | Consolation | Symbolism | References | Table of Contents | Home |
The concept of energy reference states in thermodynamics is deceptively simple in that it is often misapplied. Several examples from the literature are used to illustrate some of these difficulties.
| Introduction | Example 1 | Example 2 | Example 3 | Other Caveats | Consolation | Symbolism | References | Table of Contents | Home |
Teaching graduate courses in chemical engineering thermodynamics for several hundred students from a variety of backgrounds over the past forty years has made it evident that their comprehension of the concept of energy reference states is somewhat superficial. This circumstance is not surprising; a survey of over one hundred popular textbooks in thermodynamics for engineers and scientists revealed that, aside from a few noteworthy exceptions (discussed later), an adequate discussion of the significance of reference states is not presented. To emphasize that this subject matter is not trivial, three examples from the literature will be discussed since they illustrate some of the subtleties in reference states.
| Introduction | Example 1 | Example 2 | Example 3 | Other Caveats | Consolation | Symbolism | References | Table of Contents | Home |
Emmons (1980) makes the statement: "For ideal gases of a constant specific heat, the energy per unit volume is independent of the temperature at a given pressure". In correspondence with him, Emmons produced an anticipated response, namely: "Energy per unit volume,
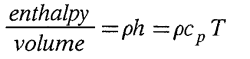
which is proportional to pressure and independent of temperature." The fallacy in his derivation lies in ignoring the integration constant. Since for an ideal gas d h º c p d T , then h = c p T + k. (Of course, if h is arbitrarily assigned a value of zero at T = 0, then the integration constant does vanish.) It is interesting to note that 13 years earlier Sommerfeld (1967) had criticized Emden (1938) for arriving at a similar conclusion (that the enthalpy per unit volume of an ideal gas was independent of temperature) as a result of having ignored the constant of integration.
The Emmons problem was referred for comment to several colleagues who regularly teach thermodynamics; two of the responses were substantive enough to merit reproduction here. Dr. S.R. Gollahalli, Professor of Mechanical Engineering at the University of Oklahoma, submitted the following:
For ideal gases,
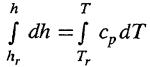
where subscript " r " implies any arbitrary value assigned to an arbitrary reference temperature.
For a given pressure P , let P = P r , so that P = r R T = P r = r r R T r .
Then
and
where g = c p / c v . (Since c p — c v = R , then c p / r B = g / g — 1). Note that in general the enthalpy per unit volume (rh) depends on T except for arbitrarily assumed values of
or
either of which is equivalent to designating (h r — c p T r ) equal to zero or alternatively, as Emmons did, setting h r = 0 for T r = 0 .
Dr. S.D. Christian, Professor of
Proc. Okla. Acad. Sci. 66:43-48 (1986)
{Page 44}
Chemistry at the University of Oklahoma, presented this analysis:
where r B is the specific gas constant.
Thus, H / V will be independent of temperature only if h = c p T which, as before, is equivalent to setting ( h r — c p T r ) = 0. Christian then adds: "Now there is nothing in thermodynamics to give us an absolute value of ' H ' . . . One cannot say how H varies in a given volume if matter is added or removed from that volume." (Note that the left side of the above equation can have a non-zero variation with temperature only if the mass varies since P and V are constant.)
To summarize, Emmons' conclusion that the enthalpy per unit volume is independent of temperature is simply an artifact of having arbitrarily defined the reference state in a particular way. (Caution must always be exercised when energy per unit volume, such as rh , is introduced for convenience in the transport equation in order to avoid analogous problems.)
| Introduction | Example 1 | Example 2 | Example 3 | Other Caveats | Consolation | Symbolism | References | Table of Contents | Home |
The previous reference to Emden was in connection with his analysis of the change in total internal energy accompanying the heating of the air in a room during which some of the room air is allowed to escape into the outdoors in order to maintain the room pressure constant. For this part of the analysis neither Emden nor Somerfeld manifested any reservations; in fact they failed to recognize that they were dealing with an open system since air had to escape from the room which was maintained at a constant pressure and volume during heating. Essentially, their analysis was based on a closed system analysis; for example:
For an ideal gas
The first term in brackets represents a measureable physical quantity, whereas the second term in brackets does not because it can take on any value depending on an arbitrary assignment of values for u r and T r . (As noted in the previous example, if u r = 0 and T r = 0, or if u r = c V T r , then the second term in brackets becomes ( m 2 — m 1 ) c V T 2 which is calculable but still meaningless in this context.)
Now if the great thermodynamicists Emden and Sommerfeld overlooked this question, then it is not unreasonable if generations of instructors and students who succeeded them might do likewise. As a matter of fact, after numerous books on thermodynamics were consulted, only two addressed this particular problem. Bridgman (1943), in the course of his profound discussion on the search for an absolute energy based on his meticulous analysis of energy as a function of a state couple rather than as a point function, concludes on page 84: "In any event it means nothing to ask what is the difference of energy between one and two grams of iron." Kestin (1966) in Volume 1, pages 157-160, by means of a specific example and supporting equations, does not leave any reason to question his conclusion: "No physical meaning can be attached to the difference between the energies of two different systems, even if the two systems merely represent different masses of the same homogeneous substance." Nevertheless, this misconception continues to propagate. For example, Modell and Reid (1983) ask for the amount of heat that would have to be added to a tank from which helium is escaping in order to keep the total energy of the gas remaining in the tank constant. Since the internal energy does not have an absolute value, then what is implied is that D U = 0 for the mass remaining in the tank at any instant. However, since the mass is changing continuously, D U is physically meaningless as explained in the preceeding paragraph. Also, as a consequence of setting D U = 0, the first law energy balance for this problem would reduce to an equality between heat transferred and the enthalpy carried out of the tank by the mass leaving. Since the numerical magnitude of the enthalpy depends on the reference state, the heat transferred would be dependent likewise,
{Page 45}
which of course is meaningless.
| Introduction | Example 1 | Example 2 | Example 3 | Other Caveats | Consolation | Symbolism | References | Table of Contents | Home |
This third example raises the question: Does the open system energy balance apply if expressed in terms of relative enthalpies and internal energies? Fredrickson (1983) appears to be the first to discuss this specific question in the published literature. Briefly, consider an open system (single substance) which for simplicity is assumed to be free of kinetic and potential energy effects.

Fredrickson (1983, page 65) states that the enthalpies, H, and internal energy, U, in Equation 2 are absolute values. He does not, however, explain what he means by absolute internal energy or enthalpy. The concept of absolute energy is in reality inconsistent with the first law energy balance which provides the operational definition for energy. Bridgman (1943) elaborates via an enlightening discourse on this subject. Notwithstanding, Fredrickson's development, including his embodiment of absolute energy terminology, runs briefly as follows:
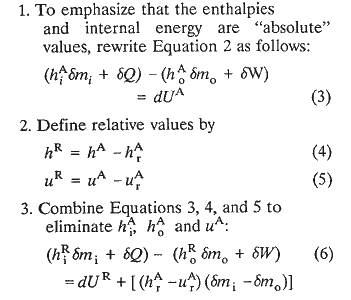
This resulting equation in terms of relative energies would be of the same form as Equation 3 if the reference states are so chosen that
in which case the term in brackets on the right of Equation 6 would vanish. However, since by immutable definition
and since in general Pv -- particularly for gases -- is not zero, Fredrickson concludes that the reference state for enthalpy must be different from the reference state for internal energy. For example, for an ideal gas: h = u + r B T , and if h r is assigned a value of zero at ( T r ) H, then (u) will have a value of [ - r B ( T r ) H ] at (T r ) H. Accordingly u will have a zero value at a temperature other than (T r ) H. This temperature, (T r ) U, can be related to (T r ) H by noting that so that
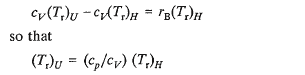
In other words, the reference state temperature for enthalpy must be different (but related in a particular way) from the reference state temperature for internal energy because, for all practical purposes, Fredrickson imposes the requirement that a zero (or prescribed) value must be assigned to a property at its particular reference state. Furthermore, his conclusion can be misleading in that there is an inference of the necessity for having different reference state temperatures for enthalpy and internal energy in the same equation. In reality, it is more conventional to assign an arbitrary numerical value to either enthalpy or internal energy -- but not both -- at one reference state temperature and to let Equation 7 determine the corresponding value of u (or h) at this temperature.
It is interesting to note that Kestin (1966, page 234 of Volume 1), in connection with his discussion on assignment of reference states for constructing tables of thermodynamic properties, cautions that it is not permissible to assume that both internal energy and enthalpy simultaneously have values of zero in the reference state. Kestin, of course, assumes that the same reference state is selected for both internal
{Page 46}
energy and enthalpy. Although Kestin (1966, Volumes 1 and 2) discussed at length "normalization of additive constants" and Planck (1948) emphasized the importance of additive constants in assigning reference states, neither of them addressed the specific question raised by Fredrickson on whether the general energy balance equation remained operative if expressed in terms of relative internal energies or enthalpies.
Going back to Fredrickson's development, if the same reference state (T and P) had been specified for internal energy and enthalpy, then a parallel derivation could be made. If Equations 4 and 5 are replaced by relationships which are consistent with the defining Equation 7, then

Since both pressure and volume are "absolute" quantities,
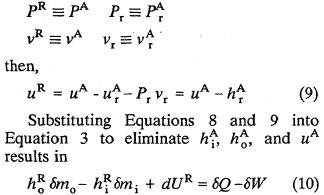
It is obvious that this form of Equation 10, in terms of "relative" energies, is identical to Equation 3 in terms of "hypothetical absolute" energies.
Aside from retaining the untenable terminology of absolute energy, the foregoing derivation is needlessly circumventive. A more direct approach is simply: Rewrite Equation 3
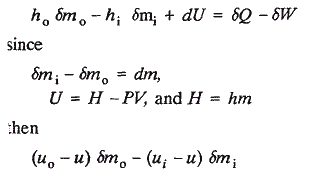
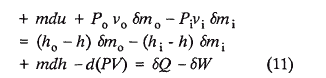
None of the quantities in the above equation is dependent on the selection of reference states. Therefore the open system energy balance remains valid even though values for "relative internal energies or enthalpies" are employed. Note in this derivation the asignment of reference states and numerical values for enthalpy vis-a-vis energy in the reference states doesn't even have to be considered. Equation 11 is an open system equivalent to the closed system energy balance. It clearly portrays that the first law concerns itself only with differences in energy, and the concept of absolute energies does not serve any purpose.
| Introduction | Example 1 | Example 2 | Example 3 | Other Caveats | Consolation | Symbolism | References | Table of Contents | Home |
The foregoing three examples focused on problems with reference states which in the final analyses were associated with the analyses of systems of variable mass. However, similar problems occur in calculating changes in the energy functions, a and g, for systems of constant mass undergoing a change in temperature. For example, for a unit mass,
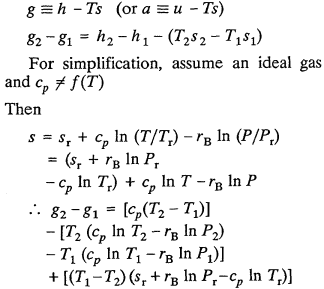
Note that the change in the Gibbs free energy per unit mass depends on the reference state chosen (except where s r = c p ln T r - r B ln P r ). Thus, it would appear that D g (or D a ) is physically meaningless except for isothermal processes. However, it is customary (but not necessary for the third
{Page 47}
law of thermodynamics) to assign a zero value to entropy at the absolute zero of temperature (Kestin, 1966). The consequence, then, is that entropy, by convention, can be assigned a pseudo absolute value at any specified temperature and pressure. For example, in the case of an ideal gas,
Values of "absolute" entropy have been calculated and tabulated for many substances at 298.15 K and the hypothetical ideal gas state of one atmosphere pressure. Then
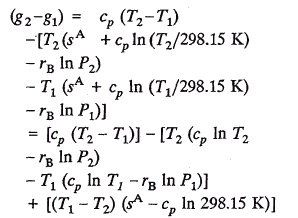
Thus, for non-isothermal processes, pseudo absolute entropies should-for the sake of consistency-be used in calculating changes in g (or a) even if the mass remains constant; otherwise, numerical values for D a and D g will "float" with the arbitrary reference state chosen.
A related problem arises when applying the Gibbs equation. For an open system free from chemical, electrical, magnetic, etc. effects:
d U = T d S - P d V + g d m
Integration yields on the left, U 2 - U 1 or u 2 m 2 - u 1 m 1 . If m 2 is different from m 1, then the difference, U 2 - U 1, is physically meaningless as discussed previously in Example II. Such difficulties can be avoided by restricting use of the Gibbs equation to a fixed or unit mass of homogeneous material, whereupon g d m drops out and
d U = T d s - P d V
In this form the Gibbs equation is simply a statement of the relationship among properties of a unit mass of matter irrespective of whether it is part of an open or closed system. This generalization follows immediately because whether a system is open or closed is purely the result of an arbitrary definition by the observer. Finally, even though the Gibbs equation is a direct result of combining the first and second laws, its principal utility in the operational sense is as a relationship among properties rather than as a combined law per se.
| Introduction | Example 1 | Example 2 | Example 3 | Other Caveats | Consolation | Symbolism | References | Table of Contents | Home |
The concept of reference states only sounds simple; in reality it can be insidious. Note that the foregoing addressed only pure substances. For multi-component systems in which concentration changes have to be considered, calculation of energy changes becomes much more elusive, particularly when having to devise "standard states" which frequently don't seem to have anything standard about them. But, take heart -- even if you err you can still be in good company!
| Introduction | Example 1 | Example 2 | Example 3 | Other Caveats | Consolation | Symbolism | References | Table of Contents | Home |
| specific | = | divided by mass; per unit mass |
| molar | = | divided by amount of substance; per mole |
| m | = | mass |
| v | = | V / m = specific volume |
| r | = | m / V = density |
| u | = | U / m = specific internal energy |
| h | = | H / m = specific enthalpy |
| s | = | S / m = specific entropy |
| g | = | G / m = specific Gibbs free energy |
| c p | = | constant-pressure specific heat capacity |
| c v | = | constant-volume specific heat capacity |
| g | = | c p / c v |
| T | = | thermodynamic temperature |
| P | = | pressure (absolute) |
| M B | = | molar mass of substance B, g / mol |
| R | = | universal molar gas constant |
| r B | = | R / MB = specific gas constant for substance B |
| Q | = | heat transferred |
{Page 48}
| W | = | work transferred | subscripts |
| r | = | reference |
| i | = | input |
| o | = | output | superscripts |
| A | = | absolute |
| R | = | relative |
| Introduction | Example 1 | Example 2 | Example 3 | Other Caveats | Consolation | Symbolism | References | Table of Contents | Home |
1. P.W. Bridgman, The Nature of Thermodynamics, Harvard University Press, Cambridge, 1943, p. 84.
2. Robert Emden, Nature 141, 908 (1938).
3. Howard W. Emmons, Ann. Rev. Fluid Mechanics 12, 227 (1980).
4. A.G. Fredrickson, Chem. Eng. Education 17(2), 64-9 (1983).
5. Joseph Kestin, A Course in Thermodynamics, Vol. 1, Blaisdell Publishing Co., Waltham, MA, 1966, p. 157 ff and p. 233 ff and Vol. 2, p. 356 ff.
6. M. Modell and R.C. Reid, Thermodynamics and Its Applications, Prentice-Hall Inc., Englewood Cliffs, NJ, 1983, p. 117, Problem 5.9.
7. Max Planck, Treatise on Thermodynamics, 3rd ed., Dover Publications, NY, 1945, p. 123.
8. Arnold Sommerfeld, Thermodynamics and Statistical Mechanics, Lectures on Theoretical Physics, Vol. 5, Academic Press, London, 1967.